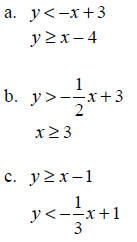Solving Systems of Linear Equations
Problem Solving Using Systems of Equations
Learning Objectives:
1. Solve problems using linear systems.
Examples:
Use variables to represent unknown quantities. Write a: Let x = and y =
statement for each problem.(Do not solve).
1. The sum of two numbers is 14. One number is six
times larger than the other. Find the two numbers.
2. Three pairs of socks and two pairs of mitten cost $42. One pair of the
same kind of socks and four pair of the mittens cost $24. Find out how much one
pair of socks and one pair of mittens cost.
3. John has $5 bills and $10 bills in his wallet. He has a total of $80.
He has twice as many $5 bills as $10 bills. How many $5 bills and how many $10
bills does he have?
Now, for problems 4 – 6, write a system of equations that
models the conditions of each problem.
(Do not solve).
4.
5.
6.
Solve each of the following using a system of equations
7. The sum of two numbers is 11. The second number
is 1 less than twice the first number. Find the two numbers.
8. Alexis has $1.65 in nickels and quarters. She has 9 coins altogether.
How many coins of each kind does she have?
9. Paul invested $12,000 in two accounts. One account paid 4% interest and
one account paid
5% interest. At the end of the year his money had earned $560 in interest. How
much did he
invest in each account?
10. A department store receives 2 shipments of bud vases and picture
frames. The first shipment
of 5 bud vases and 4 picture frames costs $62. The second shipment of 10 bud
vases and 3
picture frames cost $84. Find the cost of a vase and a picture frame.
Teaching Notes:
• Stress the importance of reading the problem
several times before beginning. Reading aloud really helps.
• Have students write a Let x= and y = statements for each word problem
before trying to write the system of equations.
• Help students look at the system they have created and determine which
method of solving will work best.
• Remind students to make sure their answers make sense for the given
situation.
• Try to build confidence with word problems.
Answers:
1. Let x = one number; let y = the other number.
2. Let x = cost of 1 pair of socks; let y = cost of 1 pair of mittens.
3. Let x = number of $5 bills; let y = number of $10 bills
4.
x + y = 14
x = 6y
5.
3x + 2y = 42
x + 4y = 24
6.
5x + 10y = 80
x = 2y
7. The numbers are 4 and 7
8. 3 nickels, 6 quarters
9. $4000 invested @ 4% and $8000 invested @ 5%
10. bud vases $6, picture frames $8
Systems of Linear Inequalities
Learning Objectives:
1. Use mathematical models involving systems of linear inequalities.
2. Graph the solution sets of systems of linear inequalities.
Examples:
1. Graph the solution set of each system.
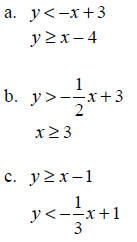
2. Name one point that is a solution for each system of
linear inequalities in examples
1a, 1b, and 1c.

Teaching Notes:
• When the inequality symbol is > or <, the line
should be dashed (- - - - -).
• When the inequality symbol is ≥ or ≤, the line should be solid (
_______).
• When graphing inequalities, it is easy to see the overlap of the graphs
if different colored
pencils are used to graph each inequality.
Answers:
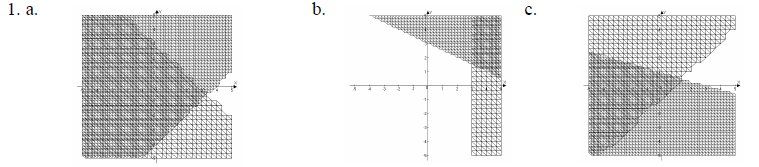
2.
a. Answers will vary
b. Answers will vary
c. Answers will vary
|